Maths & Music #1: Maths in music, the music of maths: reflections on "mathemusical dynamics", by Moreno Andreatta

This is a question that would no doubt have made scientists and professional musicians smile just thirty or so years ago, when music was often seen as entertainment and maths as a discipline too rigid to play a role in musical creativity. Times have changed since the launch, in 2007, of the first peer-reviewed journal on the relationship between mathematics and music (the Journal of Mathematics and Music), the official organ of the Society for Mathematics and Computation in Music. A few years later, this area of research was also included in the Mathematical Subject Classification (code 00A65).

But what has changed above all is the awareness on the part of mathematicians and musicians of the existence of difficult problems posed by music and likely to advance mathematical research. At the same time, the flexibility and generality of mathematical concepts and constructs have suggested to artists the value of formalising and modelling the theoretical problems posed by music. The identification of such musical problems is the starting point for a veritable "mathematical-musical dynamic".

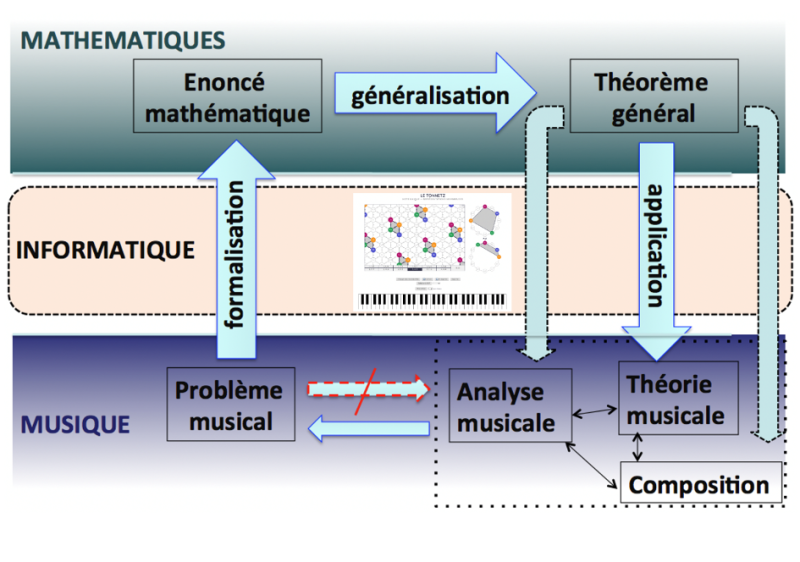
This process begins by formalising the theoretical problem using a range of tools from several branches of mathematics. This produces a mathematical statement, the first step towards a generalisation that can lead to theorems. These general results can then be applied to musical theory, analysis and composition. In this process we pass through a region that constitutes an interface between music and mathematics. This is the area of computer modelling, which sometimes consists of implementing the theoretical results obtained with a view to their musical application.

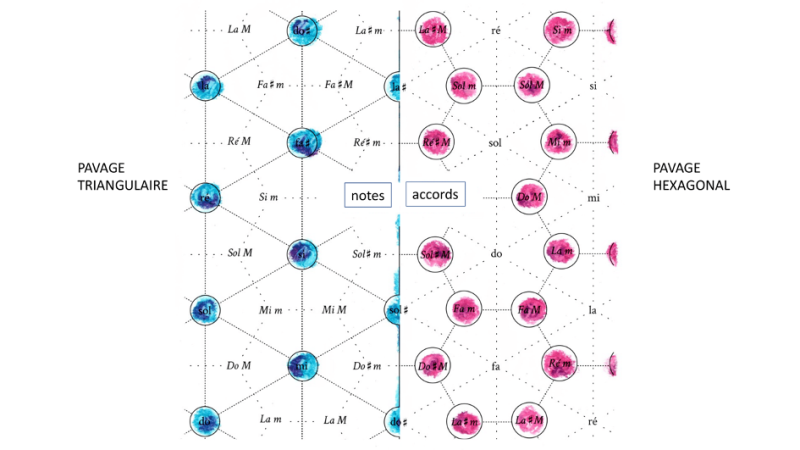
We could cite several examples of the theoretical problems underlying this dynamic, from the construction of so-called 'mosaic' musical canons to the geometric and topological representations of harmonic or rhythmic spaces. Without going into the technical aspects of the mathematical tools used, in both cases there is a fruitful link between the algebraic formalisation of the musical problem and computer modelling. This link is at the heart of the SMIR (Structural Music Information Research) project currently being carried out at Strasbourg University, , in close collaboration with the Musical Representations team at Ircam (UMR STMS). As part of this project, we have concentrated our efforts on developing open source interactive environments that can be accessed online, as they facilitate both the learning of mathematical knowledge and the application of new theoretical constructs in the field of musical creativity. This is the case, for example, with the TONNETZ environment, which allows musical notes and chords to be visualised both through representations well known to musicians (chromatic circle and cycle of fifths) and through two-dimensional spaces. These networks of notes are derived from possible decompositions of the plane by triangles along axes that correspond to the various musical intervals. This idea dates back to 18th-century music theorist and mathematician Leonardo Euler, who associated the major third and the perfect fifth, two of the most consonant intervals and the basis of the major chord, with the generating axes of the plane.

Today, this approach has been generalised to represent a musical chord in terms of the geometric space that highlights the various symmetries that make it up. Certain chord progressions can thus be formalised as spatial trajectories in the graph associated with this network of notes or Tonnetz. In this graph, we can easily move from the triangular tessellation to the corresponding hexagonal tessellation or honeycomb mesh in which the arcs linking the vertices correspond to the symmetries between chords that preserve two out of every three notes in the respective chords.

This raises the question of finding harmonic loops corresponding to spatial trajectories in the Tonnetz that allow the piece to pass through each chord once and only once, before returning to the initial point. In music, this is the equivalent of the knight problem in chess, which consists of finding a path that allows the piece to pass through all the squares on the board once and only once before returning to the starting point. In music, such a process is the basis of the so-called 'Hamiltonian' songs, songs in which you can modulate in all twenty-four major and minor keys without repeating the same type of chord twice. This is far beyond the three or four chords that make up most of the hits we are used to hearing. There's nothing artificial about the end result, as the reader can see by listening, for example, to the Hamiltonian song 'Le blé en herbe' by Polo Lamy, available on my Youtube channel.

But, beyond specialists in mathematics or music, is it possible to pass on mathémusicaux (mathematical and musical) knowledge to a public with no in-depth knowledge of either maths or music? This is the challenge we are now trying to meet through the LaMaMu project, supported by the University of Strasbourg's IDEX 'Sciences et société et en territoire' and CREAA, which is rolling out a series of initiatives aimed at secondary school pupils and the general public. Educational workshops run in partnership with teachers from the French Ministry of Education's LaboMath scheme enable pupils to familiarise themselves with a number of mathematical concepts using music as a starting point. The same theoretical constructs on which the school workshops are based are also used in the Math'n Pop show, which was initially approved by the CNRS as part of its 80th anniversary celebrations.

This theatrical lecture-concert uses music to dramatise mathematical ideas and concepts, a perfect way to get the general public interested in maths. Other initiatives aimed at the general public are underway, such as the famous mathematical calendar, the 2025 edition of which will be devoted to mathemusic. Every month, this will be a valuable opportunity to discover a subject that touches on different aspects of the mathematics/music relationship, with a series of mathematical challenges that will enable readers to gradually grasp some of the ideas behind this inexhaustible mathemusical dynamic.